Edge Map

We begin by defining a non-negative edge map f(x,y) derived from the image I(x,y) having the property that it is larger near the image edges. Accordingly, we can use

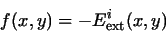$$f(x,y) = - E_{\rm ext}^i(x,y)$$ (9)

where i = 1, 2, 3, or 4. The field $\nabla f$ has vectors pointing toward the edges, but it has a narrow capture range, in general. Furthermore, in homogeneous regions, I(x,y) is constant, $\nabla f$ is zero, and therefore no information about nearby or distant edges is available.

We note that other features besides edges can be sought by redefining f(x,y) to be larger at desired features of interest, rather than edges.